Earth Rotation and Revolution
The term Earth rotation refers to the spinning of our planet on its axis. Because of rotation, the Earth's surface moves at the equator at a speed of about 467 m per second or slightly over 1675 km per hour. If you could look down at the Earth's North Pole from space you would notice that the direction of rotation is counter-clockwise (Figure 6h-1). The opposite is true if the Earth is viewed from the South Pole. One rotation takes exactly twenty-four hours and is called a mean solar day. The Earth’s rotation is responsible for the daily cycles of day and night. At any one moment in time, one half of the Earth is in sunlight, while the other half is in darkness. The edge dividing the daylight from night is called the circle of illumination. The Earth’s rotation also creates the apparent movement of the Sun across the horizon.


| Figure 6h-1: The movement of the Earth about its axis is known as rotation. The direction of this movement varies with the viewer’s position. From the North Pole the rotation appears to move in a counter-clockwise fashion. Looking down at the South Pole the Earth’s rotation appears clockwise. |
The orbit of the Earth around the Sun is called an Earth revolution. This celestial motion takes 365.26 days to complete one cycle. Further, the Earth's orbit around the Sun is not circular, but oval or elliptical (see Figure 6h-2). An elliptical orbit causes the Earth's distance from the Sun to vary over a year. Yet, this phenomenon is not responsible for the Earth’s seasons! This variation in the distance from the Sun causes the amount of solar radiation received by the Earth to annually vary by about 6%. Figure 6h-2 illustrates the positions in the Earth’s revolution where it is closest and farthest from the Sun. On January 3, perihelion, the Earth is closest to the Sun (147.3 million km). The Earth is farthest from the Sun on July 4, or aphelion (152.1 million km). The average distance of the Earth from the Sun over a one-year period is about 149.6 million km.
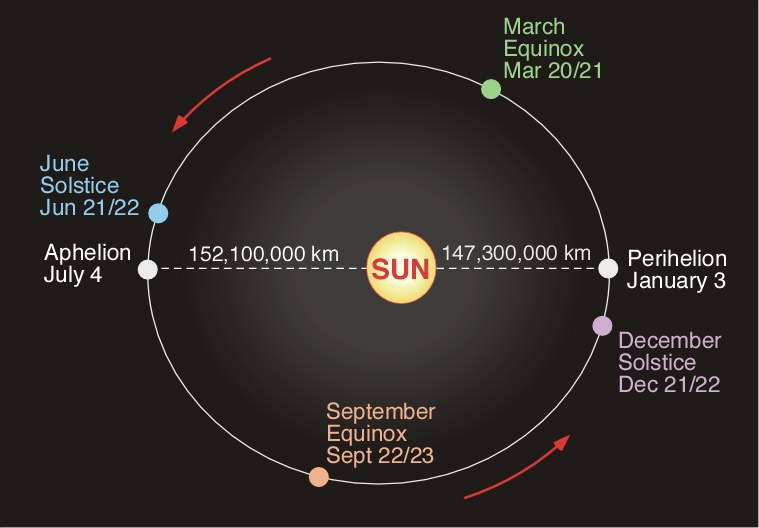
| Figure 6h-2: Position of the equinoxes, solstices, aphelion, and perihelion relative to the Earth's orbit around the Sun. The equinoxes and solstices should be 90º apart in the ecliptic plane. |
Tilt of the Earth's Axis
The ecliptic plane can be defined as a two-dimensional flat surface that geometrically intersects the Earth's orbital path around the Sun. On this plane, the Earth's axis is not at right angles to this surface, but inclined at an angle of about 23.5° from the perpendicular. Figure 6h-3 shows a side view of the Earth in its orbit about the Sun on four important dates: June solstice, September equinox, December solstice, and March equinox. Note that the angle of the Earth's axis in relation to the ecliptic plane and the North Star on these four dates remains unchanged. Yet, the relative position of the Earth's axis to the Sun does change during this cycle. This circumstance is responsible for the annual changes in the height of the Sun above the horizon. It also causes the seasons, by controlling the intensity and duration of sunlight received by locations on the Earth. Figure 6h-4 shows an overhead view of this same phenomenon. In this view, we can see how the circle of illumination changes its position on the Earth’s surface. During the two equinoxes, the circle of illumination cuts through the North Pole and the South Pole. On the June solstice, the circle of illumination is tangent to the Arctic Circle (66.5° N) and the region above this latitude receives 24 hours of daylight. The Arctic Circle is in 24 hours of darkness during the December solstice.

| Figure 6h-3:The Earth’s rotational axis is tilted 23.5° from the red line drawn perpendicular to the ecliptic plane. This tilt remains the same anywhere along the Earth’s orbit around the Sun. Seasons are appropriate only for the Northern Hemisphere. |
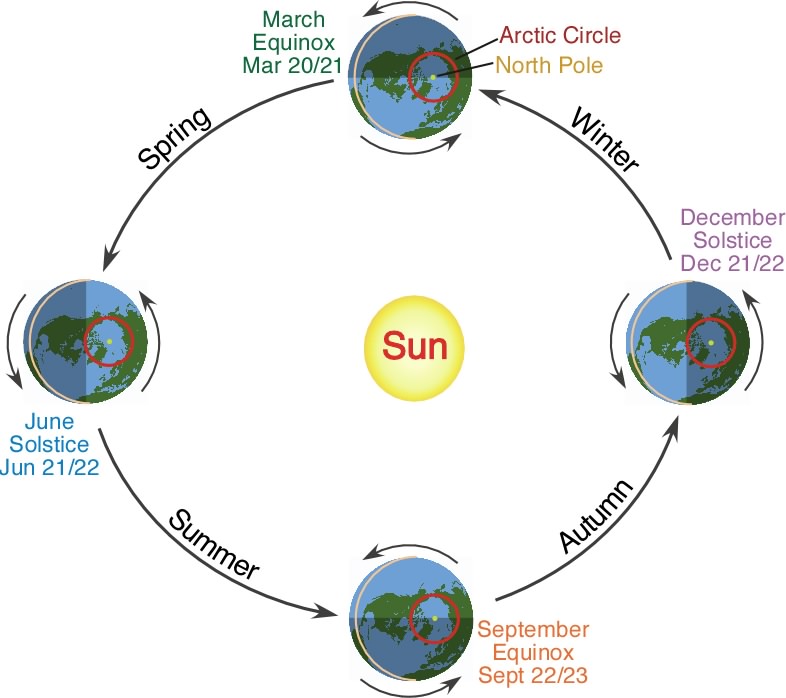
| Figure 6h-4: Annual change in the position of the Earth in its revolution around the Sun. In this graphic, we are viewing the Earth from a position in space that is above the North Pole (yellow dot) at the summer solstice, the winter solstice, and the two equinoxes. Note how the position of the North Pole on the Earth's surface does not change. However, its position relative to the Sun does change and this shift is responsible for the seasons. The red circle on each of the Earths represents the Arctic Circle (66.5 degrees N). During the June solstice, the area above the Arctic Circle is experiencing 24 hours of daylight because the North Pole is tilted 23.5 degrees toward the Sun. The Arctic Circle experiences 24 hours of night when the North Pole is tilted 23.5 degrees away from the Sun in the December solstice. During the two equinoxes, the circle of illumination cuts through the polar axis and all locations on the Earth experience 12 hours of day and night. Seasons are appropriate only for the Northern Hemisphere. |
On June 21 or 22 the Earth is positioned in its orbit so that the North Pole is leaning 23.5° toward the Sun (Figures 6h-3, 6h-4, 6h-5 and see animation - Figure 6h-7). During the June solstice (also called the summer solstice in the Northern Hemisphere), all locations north of the equator have day lengths greater than twelve hours, while all locations south of the equator have day lengths less than twelve hours (see Table 6h-2). On December 21 or 22 the Earth is positioned so that the South Pole is leaning 23.5 degrees toward the Sun (Figures 6h-3, 6h-4, 6h-5 and see animation - Figure 6h-8). During the December solstice (also called the winter solstice in the Northern Hemisphere), all locations north of the equator have day lengths less than twelve hours, while all locations south of the equator have day lengths exceeding twelve hours (see Table 6h-2).

| Figure 6h-5: During the June solstice the Earth's North Pole is tilted 23.5 degrees towards the Sun relative to the circle of illumination. This phenomenon keeps all places above a latitude of 66.5 degrees N in 24 hours of sunlight, while locations below a latitude of 66.5 degrees S are in darkness. The North Pole is tilted 23.5 degrees away from the Sun relative to the circle of illumination during the December solstice. On this date, all places above a latitude of 66.5 degrees N are now in darkness, while locations below a latitude of 66.5 degrees S receive 24 hours of daylight. |
On September 22 or 23, also called the autumnal equinox in the Northern Hemisphere, neither pole is tilted toward or away from the Sun (Figures 6h-3, 6h-4, 6h-6 and see animation - Figure 6h-9). In the Northern Hemisphere, March 20 or 21 marks the arrival of the vernal equinox or spring when once again the poles are not tilted toward or away from the Sun. Day lengths on both of these days, regardless of latitude, are exactly 12 hours.
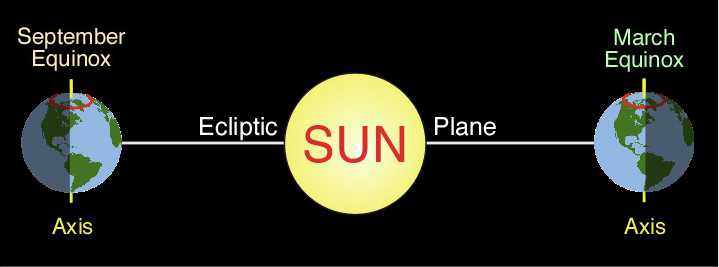
| Figure 6h-6: During the equinoxes, the axis of the Earth is not tilted toward or away from the Sun and the circle of illumination cuts through the poles. This situation does not suggest that the 23.5 degree tilt of the Earth no longer exists. The vantage point of this graphic shows that the Earth's axis is inclined 23.5 degrees toward the viewer for both dates (see Figures 6h-3 and 6h-4). The red circles shown in the graphic are the Arctic Circle. |
Axis Tilt and Solar Altitude
The annual change in the relative position of the Earth's axis in relationship to the Sun causes the height of the Sun or solar altitude to vary in our skies. Solar altitude is normally measured from either the southern or northern point along the horizon and begins at zero degrees. Maximum solar altitude occurs when the Sun is directly overhead and has a value of 90°. The total variation in maximum solar altitude for any location on the Earth over a one-year period is 47° (Earth’s tilt 23.5° x 2 = 47°). This variation is due to the annual changes in the relative position of the Earth to the Sun. At 50 degrees North, maximum solar altitude varies from 63.5 degrees on the June solstice to 16.5 degrees on the December solstice (Figure 6h-10). Maximum solar height at the equator goes from 66.5 degrees above the northern end of the horizon during the June solstice, to directly overhead on the September equinox, and then down to 66.5 degrees above the southern end of the horizon during the December solstice (Figure 6h-11).

| Figure 6h-10: Variations in solar altitude at solar noon for 50 degrees North during the June solstice, equinox, and December solstice. |

| Figure 6h-11: Variations in solar altitude at solar noon for the equator during the June solstice, equinox, and December solstice. |
The location on the Earth where the Sun is directly overhead at solar noon is known as the subsolar point. The subsolar point occurs on the equator during the two equinoxes (Figures 6h-11 and 6h-12). On these dates, the equator is lined up with the ecliptic plane and the poles are in line with the circle of illumination (Figure 6h-6). During the summer solstice, the subsolar point moves to the Tropic of Cancer (23.5° N) because at this time the North Pole is inclined 23.5° toward the Sun (Figures 6h-12 and 6h-13). Figure 6h-13 shows how the subsolar point gradually changes from one day to the next over a period of one-year. Note that on this graph, the subsolar point is located at the Tropic of Capricorn (23.5° S) during the December solstice when the South Pole is angled 23.5° toward the Sun (Figure 6h-5).

| Figure 6h-12: Relationship of maximum Sun height to latitude for the equinox (left) and June solstice (right). The red values on the right of the globes are maximum solar altitudes at solar noon. Black numbers on the left indicate the location of the Equator, Tropic of Cancer (23.5 degrees N), Tropic of Capricorn (23.5 degrees S), Arctic Circle (66.5 degrees N), and the Antarctic Circle (66.5 degrees S). The location of the North and South Poles are also identified. During the equinox, the equator is the location on the Earth with a Sun angle of 90 degrees for solar noon. Note how maximum Sun height declines with latitude as you move away from the Equator. For each degree of latitude traveled maximum Sun height decreases by the same amount. At equinox, you can also calculate the noon angle by subtracting the location's latitude from 90. During the summer solstice, the Sun is now directly overhead at the Tropic of Cancer. All locations above this location have maximum Sun heights that are 23.5 degrees higher from the equinox situation. Places above the Arctic Circle are in 24 hours of daylight. Below the Tropic of Cancer the noon angle of the Sun drops one degree in height for each degree of latitude traveled. At the Antarctic Circle, maximum Sun height becomes 0 degrees and locations south of this point on the Earth are in 24 hours of darkness. |

| Figure 6h-13: Angle of the Sun’s declination and latitude of the subsolar point throughout the year. Seasons are for the Northern Hemisphere. |
The following table describes the changes in solar altitude at solar noon for the two solstices and equinoxes. All measurements are in degrees (horizon has 180 degrees from True North to True South) and are measured from either True North or True South (whatever is closer).
| Table 6h-1: Maximum Sun altitudes for selected latitudes during the two solstices and equinoxes. |
Location's Latitude |
March Equinox March 20/21 |
June Solstice June 21/22 |
September Equinox September 22/23 |
December Solstice December 21/22 |
| 90 N | 0 degrees | 23.5 degrees | 0 degrees | - 23.5 degrees |
| 70 N | 20 degrees | 43.5 degrees | 20 degrees | -3.5 degrees |
| 66.5 N | 23.5 degrees | 47 degrees | 23.5 degrees | 0 degrees |
| 60 N | 30 degrees | 53.5 degrees | 30 degrees | 6.5 degrees |
| 50 N | 40 degrees | 63.5 degrees | 40 degrees | 16.5 degrees |
| 23.5 N | 66.5 degrees | 90 degrees | 66.5 degrees | 43 degrees |
| 0 degrees | 90 degrees | 66.5 degrees | 90 degrees | 66.5 degrees |
| 23.5 S | 66.5 degrees | 43 degrees | 66.5 degrees | 90 degrees |
| 50 S | 40 degrees | 16.5 degrees | 40 degrees | 63.5 degrees |
| 60 S | 30 degrees | 6.5 degrees | 30 degrees | 53.5 degrees |
| 66.5 S | 23.5 degrees | 0 degrees | 23.5 degrees | 47 degrees |
| 70 S | 20 degrees | -3.5 degrees | 20 degrees | 43.5 degrees |
| 90 S | 0 degrees | - 23.5 degrees | 0 degrees | 23.5 degrees |
The following links show graphical illustrations of the annual movements of the Sun in our skies for selected latitudes. In these illustrations, solar angles are measured from both True North and True South for solar noon.
| 90 N | 66.5 N | 50 N | 23.5 N | Equator (0) | 23.5 S | 50 S | 66.5 S | 90 S |
Finally, the altitude of the Sun at solar noon can also be calculated with the following simple equation:
Altitude A = 90 - Latitude L +/- Declination D
In this equation, L is the latitude of the location in degrees and D is the declination. The equation is simplified to A = 90 - L if Sun angle determinations are being made for either equinox date. If the Sun angle determination is for a solstice date, declination (D) is added to latitude (L) if the location is experiencing summer (northern latitudes = June solstice; southern latitudes = December solstice) and subtracted from latitude (L) if the location is experiencing winter (northern latitudes = December solstice; southern latitudes = June solstice). All answers from this equation are given relative to True North for southern latitudes and True South for northern latitudes. For our purposes only the declinations of the two solstices and two equinoxes are important. These values are: June solstice D=23.5, December solstice D=-23.5, March equinox D=0, and September equinox D=0. When using the above equation in tropical latitudes, Sun altitude values greater than 90 degrees may occur for some calculations. When this occurs, the noonday Sun is actually behind you when looking towards the equator. Under these circumstances, Sun altitude should be recalculated as follows: